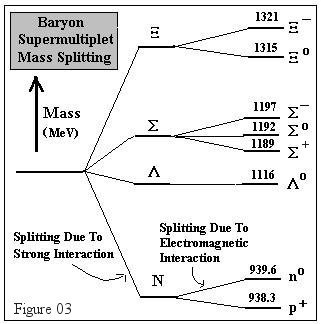
 |
A group is defined as a collection of elements (a set), which are labelled a, b, c, ... and so on, and which are related to one another by the following rules:
- If a and b are both members of the group G, then their product, c = ab is also a member of the group G.
- The process is associative, i.e., a(bc) = (ab)c.
- There must be an element, called the unit element and usually denoted by e, defined so that ae = a, be = b, and so on for all elements in the group.
- Each element has an inverse, written as a-1, b-1 and so on, defined so that aa-1 = e and so on.
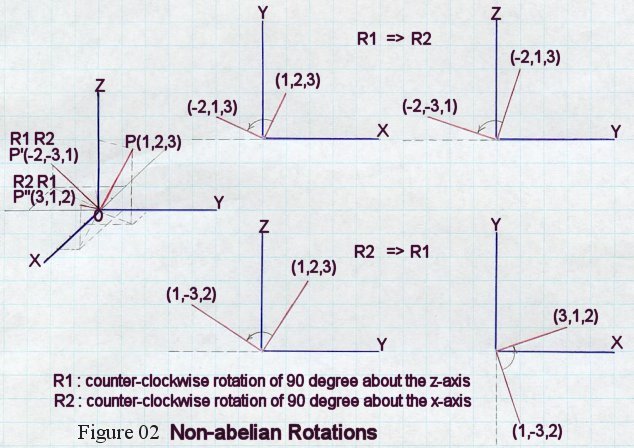
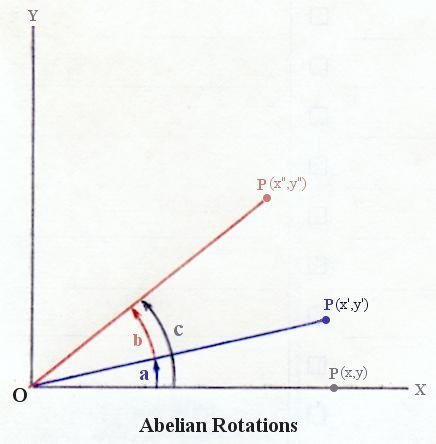
A group for which ab = ba is an Abelian group. The set of ordinary integer numbers ( ... -3, -2, -1, 0, 1, 2, 3, ...) under "addition" is a simple example of an Abelian group, where "0" is the unit element and the inverse is the same number with opposite sign.
|