|
Pythagoras There is geometry in the
humming of the strings
Pythagoras From Egypt we move across the Mediterranean Sea to the Greek island of Samos, the birthplace of Pythagoras, whose ideas dominate most of the material in this course. We'll introduce Pythagoras and his secret society of the Pythagoreans. We'll look at the Pythagoreans' ideas about numbers, as a prelude to our next unit on number symbolism. Finally, we'll introduce a new idea that will be recurring theme throughout this course, the musical ratios, which will reappear in discussions of the architecture of the Renaissance. Our main link between Egypt and Greece seems to be Thales c 640-550 BC, father of Greek mathematics, astronomy, and Philosophy, and was one of the Seven Sages of Greece. A rich merchant, his duties as a merchant took him to Egypt, and so became one of the main sources of Egyptian mathematical information in Greece. It was Thales advised his student to visit Egypt, and that student was Pythagoras. Raphael's School of Athens Pythagoras is shown in this famous painting, done by Raphael in 1510-11, which also shows most of the Greek philosophers. Socrates sprawls on the steps at their feet, the hemlock cup nearby. His student Plato the idealist is on the left, pointing upwards to divine inspiration. He holds his Timaeus, a book we'll talk about soon. Plato's student Aristotle, the man of good sense, stands next to him. He is holding his Ethics in one hand and holding out the other in a gesture of moderation, the golden mean. Euclid is shown with compass, lower right. He is the Greek mathematician whose Elements we'll mention often.
Finally, we see Pythagoras (582?-500? BC), Greek philosopher and mathematician, in the lower-left corner. The Pythagoreans Pythagoras was born in Ionia on the island of Sámos, and eventually settled in Crotone, a Dorian Greek colony in southern Italy, in 529 B.C.E. There he lectured in philosophy and mathematics. He started an academy which gradually formed into a society or brotherhood called the Order of the Pythagoreans. Disciplines of the Pythagoreans included:
We see here the roots of later monastic orders. For badges and symbols, the Pythagoreans had the Sacred Tetractys and the Star Pentagram, both of which we'll talk about later. There were three degrees of membership: 1. novices or "Politics" The Pythagoreans relied on oral teaching, perhaps due to their pledge of secrecy, but their ideas were eventually committed to writing. Pythagoras' philosophy is known only through the work of his disciples, and it's impossible to know how much of the "Pythagorean" discoveries were made by Pythagoras himself. It was the tradition of later Pythagoreans to ascribe everything to the Master himself. Pythagorean Number Symbolism The Pythagoreans adored numbers. Aristotle, in his Metaphysica, sums up the Pythagorean's attitude towards numbers. "The (Pythagoreans were) ... the first to take up mathematics ... (and) thought its principles were the principles of all things. Since, of these principles, numbers ... are the first, ... in numbers they seemed to see many resemblances to things that exist ... more than [just] air, fire and earth and water, (but things such as) justice, soul, reason, opportunity ..." The Pythagoreans knew just the positive whole numbers. Zero, negative numbers, and irrational numbers didn't exist in their system. Here are some Pythagorean ideas about numbers. Masculine and Feminine Numbers Odd numbers were considered masculine; even numbers feminine because they are weaker than the odd. When divided they have, unlike the odd, nothing in the center. Further, the odds are the master, because odd + even always give odd. And two evens can never produce an odd, while two odds produce an even. Since the birth of a son was considered more fortunate than birth of a daughter, odd numbers became associated with good luck. "The gods delight in odd numbers," wrote Virgil. 1 Monad. Point. The source of all numbers. Good, desirable, essential, indivisible. 2 Dyad. Line. Diversity, a loss of unity, the number of excess and defect. The first feminine number. Duality. 3 Triad. Plane. By virtue of the triad, unity and diversity of which it is composed are restored to harmony. The first odd, masculine number. 4 Tetrad. Solid. The first feminine square. Justice, steadfast and square. The number of the square, the elements, the seasons, ages of man, lunar phases, virtues. 5 Pentad. The masculine marriage number, uniting the first female number and the first male number by addition.
6 The first feminine marriage number, uniting 2 and 3 by
multiplication. 7 Heptad. The maiden goddess Athene, the virgin number, because 7 alone has neither factors or product. Also, a circle cannot be divided into seven parts by any known construction). 8 The first cube. 9 The first masculine square. 10 Decad. Number of fingers or toes. 27 The first masculine cube. 28 Astrologically significant as the
lunar cycle. 35 Sum of the first feminine and masculine cubes (8+27) 36 Product of the first square numbers
(4 x 9) Figured Numbers The Pythagoreans represented numbers by patterns of dots, probably a result of arranging pebbles into patterns. The resulting figures have given us the present word figures. Thus 9 pebbles can be arranged into 3 rows with 3 pebbles per row, forming a square. Similarly, 10 pebbles can be arranged into four rows, containing 1, 2, 3, and 4 pebbles per row, forming a triangle. From these they derived relationships between numbers. For example, noting that a square number can be subdivided by a diagonal line into two triangular numbers, we can say that a square number is always the sum of two triangular numbers. Thus the square number 25 is the sum of the triangular number 10 and the triangular number 15. Sacred Tetractys One particular triangular number that they especially liked was the number ten. It was called a Tetractys, meaning a set of four things, a word attributed to the Greek Mathematician and astronomer Theon (c. 100 CE). The Pythagoreans identified ten such sets. Ten Sets of Four Things
Gnomons Gnomon means carpenter's square in Greek. Its the name given to the upright stick on a sundial. For the Pythagoreans, the gnomons were the odd integers, the masculine numbers. Starting with the monad, a square number could be obtained by adding an L-shaped border, called a gnomon. Thus, the sum of the monad and any consecutive number of gnomons is a square number. 1 + 3 = 4 1 + 3 + 5 = 9 1 + 3 + 5 + 7 = 16 and so on. The Quadrivium While speaking of groups of four, we owe another one to the Pythagoreans, the division of mathematics into four groups,
giving the famous Quadrivium of knowledge, the four subjects needed for a bachelor's degree in the Middle Ages. Music of the Spheres Jubal and Pythagoras
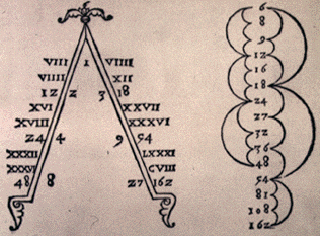
So the Pythagoreans in their love of numbers built up this elaborate number lore, but it may be that the numbers that impressed them most were those found in the musical ratios. Lets start with this frontispiece from a 1492 book on music theory. The upper left frame shows Lubal or Jubal, from the Old Testament, "father of all who play the lyre and the pipe" and 6 guys whacking on an anvil with hammers numbered 4, 6, 8, 9, 12, 16. The frames in the upper right and lower left show Pithagoras hitting bells, plucking strings under different tensions, tapping glasses filled to different lengths with water, all marked 4, 6, 8, 9, 12, 16. In each frame he sounds the ones marked 8 and 16, an interval of 1:2 called the octave, or diapason. In the lower right, he and Philolaos, another Pythagorean, blow pipes of lengths 8 and 16, again giving the octave, but Pythagoras holds pipes 9 and 12, giving the ratio 3:4, called the fourth or diatesseron while Philolaos holds 4 and 6, giving the ratio 2:3, called the fifth or diapente. They are:
These were the only intervals considered harmonious by the Greeks. The Pythagoreans supposedly found them by experimenting with a single string with a moveable bridge, and found these pleasant intervals could be expressed as the ratio of whole numbers. Pythagoras in the School of Athens
Raphael's School of Athens shows Pythagoras is explaining the musical ratios to a pupil. Notice the tablet. It shows: The words diatessaron, diapente, diapason. Under the tablet is a triangular number 10 called the sacred tetractys, that we mentioned earlier. The Harmonic Scale
This diagram from a book written in 1518 shows the famous Renaissance musical theorist Franchino Gafurio with three organ pipes and 3 strings marked 3 , 4, 6. This indicates the octave, 3 : 6 divided by the harmonic mean 4, into the fourth, 3 : 4, and the fifth, 4 : 6 or 2 : 3. The banner reads, "Harmonia est discordia concors" or Harmony is discordant concord, propounding the thesis that harmony results from two unequal intervals drawn from dissimilar proportions. The diagram shows compasses, suggesting a link between geometry and music. So What? So after experimenting with plucked strings the Pythagoreans discovered that the intervals that pleased people's ears were
and we can add the two Greek composite consonances, not mentioned before . . .
Now bear in mind that we're dealing with people that were so nuts about numbers that they made up little stories about them and arranged pebbles to make little pictures of them. Then they discovered that all the musical intervals they felt was beautiful, these five sets of ratios, were all contained in the simple numbers 1, 2, 3, 4 and that these were the very numbers in their beloved sacred tetractys that added up to the number of fingers. They must have felt they had discovered some basic laws of the universe. Quoting Aristotle again ... "[the Pythagoreans] saw that the ... ratios of musical scales were expressible in numbers [and that] .. all things seemed to be modeled on numbers, and numbers seemed to be the first things in the whole of nature, they supposed the elements of number to be the elements of all things, and the whole heaven to be a musical scale and a number." Music of the Spheres
"... and the whole heaven to be a musical scale and a number... " It seemed clear to the Pythagoreans that the distances between the planets would have the same ratios as produced harmonious sounds in a plucked string. To them, the solar system consisted of ten spheres revolving in circles about a central fire, each sphere giving off a sound the way a projectile makes a sound as it swished through the air; the closer spheres gave lower tones while the farther moved faster and gave higher pitched sounds. All combined into a beautiful harmony, the music of the spheres. This idea was picked up by Plato, who in his Republic says of the cosmos; ". . . Upon each of its circles stood a siren who was carried round with its movements, uttering the concords of a single scale," and who, in his Timaeus, describes the circles of heaven subdivided according to the musical ratios. Kepler, 20 centuries later, wrote in his Harmonice Munde (1619) says that he wishes "to erect the magnificent edifice of the harmonic system of the musical scale . . . as God, the Creator Himself, has expressed it in harmonizing the heavenly motions." And later, "I grant you that no sounds are given forth, but I affirm . . . that the movements of the planets are modulated according to harmonic proportions." Systems of Proportions based on the Musical Ratios
Slide 17-1: Villa Capra Rotunda What does this have to do with art or architecture? The idea that the same ratios that are pleasing to the ear would also be pleasing to the eye appears in the writings of Plato, Plotinus, St. Augustine, and St. Aquinas. But the most direct statement comes from the renaissance architect Leone Battista Alberti (1404-1472), "[I am] convinced of the truth of Pythagoras' saying, that Nature is sure to act consistently . . . I conclude that the same numbers by means of which the agreement of sounds affect our ears with delight are the very same which please our eyes and our minds." Alberti then gives a list of ratios permissible, which include those found by Pythagoras. We'll encounter Alberti again for he is a central figure in the development of perspective in painting. We'll also discuss another architect who used musical ratios, Andrea Palladio (1518-1580), who designed the Villa Capra Rotunda shown here.
Summary
I always wanted to make a pilgrimage to Crotone, site of the Pythagorean cult, but this is all that's there to mark their presence. Pythagoras and his followers died when their meetinghouse was torched. We'll have more on the Pythagoreans later, in particular their fondness for the star pentagram. |