 |
 |
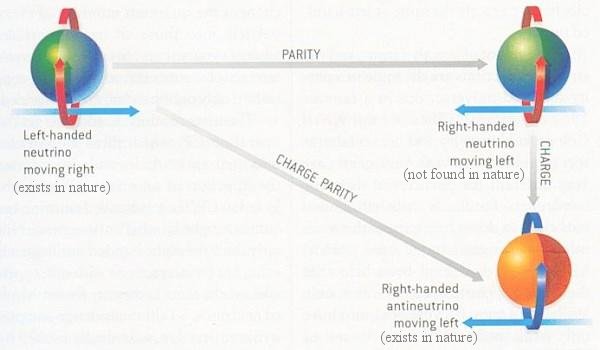
As shown in Figure 01, charge conjugation (C) reverses the sign of quantum numbers such as electric charge, changing a particle to its antiparticle. Parity (P) reverses the arrow on all vectors associated with the object. The laws of classical mechanics and electromagnetism are invariant under either of these operations, as is the strong interaction of the Standard Model. These symmetries, |
Figure 01 CP Violation |
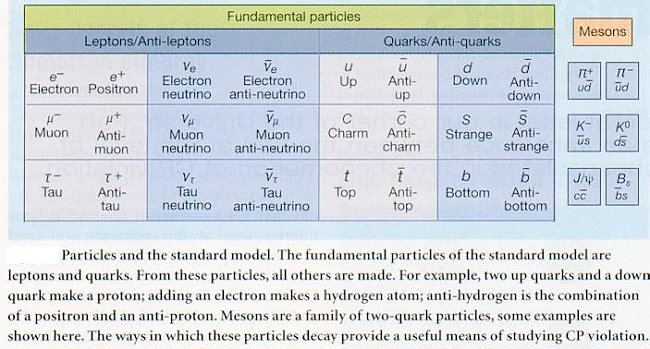
Figure 02 Elementary Part-icles [view large image] |
however, are broken in the weak interaction. For many years, it appeared that the combined operation (CP) were invariant even for weak interactions until it was shown to be otherwise in 1964. |
 |
decay into three neutral pions (also with CP=-1). The 1964 experiment by Cronin and Fitch looked for the decay products of Klong. They observed a few decays of the Klong turning into pairs of oppositely charged pions: about 1 out of a total of 500 decays. This kind of decay arrives at a final CP state that is different from the initial CP state and proves that CP symmetry was not preserved exactly by the weak interaction. |
Figure 03 Ko Meson Decays |
 |
In contrast with electric charge, which seems to come in a well-defined universal unit, each of these nine coupling constants is different. The triumph of the Standard Model is that it predicts a set of relationships between the nine elements of the CKM matrix and it predicts that they include properties that result in CP violation. The CP violation is related to the fact that the matrix elements include imaginary numbers. If we look at enough decays that involve the different matrix elements, we can see whether the relationships are true (there are only four independent parameters in the matrix). |
Figure 04 CKM Matrix |
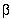 = arg(VtdVtb*/VcdVcb*), i.e., the polar angle of the complex number VtdVtb*/VcdVcb*, to determine the degree of CP-violation in Bo decay. The different modes of decay are also indicated in the diagram; D is the D meson containing a charm quark and a light quark.
= arg(VtdVtb*/VcdVcb*), i.e., the polar angle of the complex number VtdVtb*/VcdVcb*, to determine the degree of CP-violation in Bo decay. The different modes of decay are also indicated in the diagram; D is the D meson containing a charm quark and a light quark.
 |
Figure 05 Unitarity Triangle |
 have very small branching ratios and in general low efficiencies for complete reconstruction of the final state. It is therefore necessary to produce a very large sample of B mesons to perform a CP measurement. At the High Energy Accelerator Research Organization (KEK) in Japan and the Stanford Linear Accelerator Center (SLAC) in California, accelerators have been designed to produce a plentiful supply of B mesons, through specially tuned electron - positron collisions. At each facility is a detector (Belle in Japan, and BaBar in California) to pick up and study
have very small branching ratios and in general low efficiencies for complete reconstruction of the final state. It is therefore necessary to produce a very large sample of B mesons to perform a CP measurement. At the High Energy Accelerator Research Organization (KEK) in Japan and the Stanford Linear Accelerator Center (SLAC) in California, accelerators have been designed to produce a plentiful supply of B mesons, through specially tuned electron - positron collisions. At each facility is a detector (Belle in Japan, and BaBar in California) to pick up and study
 |
the decays of the many millions of B mesons created - hence these facilities are known as "B factories". Figure 06 shows the BaBar detector and the particle tracks from the B meson decay. The latest measurements yield a value of sine(2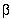 ) = 0.78 ) = 0.78  0.08, which is exactly that needed to explain the magnitude of CP violation seen in the Cronin-Fitch experiment. However, these measurements also predict a value of one leftover proton to 1018 photons (resulting from the annihilation of particles and antiparticles). It is in disagreement with observation by many orders of magnitude. 0.08, which is exactly that needed to explain the magnitude of CP violation seen in the Cronin-Fitch experiment. However, these measurements also predict a value of one leftover proton to 1018 photons (resulting from the annihilation of particles and antiparticles). It is in disagreement with observation by many orders of magnitude.
|
Figure 06 B Meson Decay |
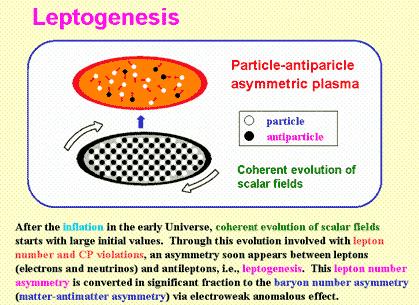 |
The disagreement with observation is now supported by three years of B meson decay data. It seems that the Standard model alone is not able to explain the phenomenon of matter-antimatter asymmetry. A new theory called leptogenesis (Figure 07) suggests that an exceptionally heavy but unstable breed of neutrino existed in the very early universe. These heavy neutrinos decayed to the second generation of neutrinos and anti-neutrinos with a bias toward the neutrinos. They then changed their masses, becoming protons/neutrons and antiprotons/antineutrons, and leading to the imbalance between matter and antimatter at the dawn of time. |
Figure 07 Leptogenesis |
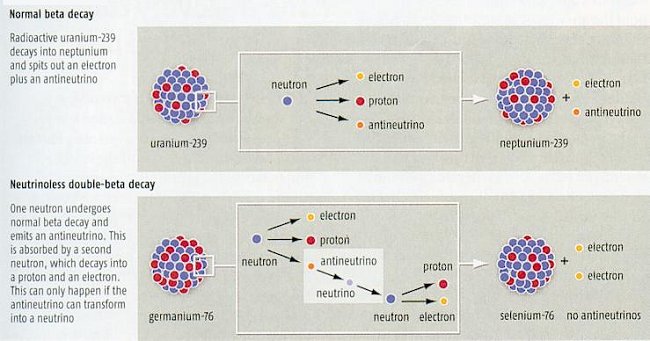 |
An experiment to look for neutrinoless double-beta decay (NDB, see Figure 08) is running since 1990 at the Gran Sasso laboratory near Rome. The claim for discovery is still in dispute. But the findings could confirm the neutrinos' changing behaviour (flip-floping between antineutrino and neutrino as shown in the lower diagram of Figure 08), and the existence of an extremely heavy form of neutrino at high temperatures (such as in the aftermath of the big bang). |
Figure 08 NDB Decay |